Complexity and beauty : the uncanny peak

Pierre Berger, last updated 14/1/2013

A figure in Philip Galanter’s Complexity and the Role of Evolutionary Art, in [Romero]prised us. This wide panoramic state of the arts is sometimes teasing by its vagueness, but no less stimulating. A surprising curve illustrates it, with a pointed peak, when, in such a universe rather statistically minded, a Gaussian bell curve would seem more abiding. :

Such a curve, with its cusp, would call for precise coordinates for this point. But nothing in the text shows how these coordinates could be computed or even approximated. On the contrary indeed, the context, more philosophical that mathematical, evokes soft and blurred lines.  But the competence of the author, as well as our own intuitions, pushed us to look around for similar constructs about complexity and esthetics.  Let us list our discoveries, coming from very different environments.

- The “effective complexity” concept is taken, says Galanter, from the physics theorist Gell-Mann (Nobel prise for the quarks theory. In his vulgarisation book [Gell-Mann,1997] we find an even rough drawing. He qualifies it himself as a simplification. But does not give answers to our above questions.

A more « bell like » figure is proposed in the same Gell-Mann book, but with no clear correlation with the precedent.

In the biosemiotics field, a suggestive figure about algorithms and complexity could combine the different approaches, according to [Abel and Trevors] :

- The « narrative interest » is defined by [Dessalles] Jean-Louis Dessalles as the difference of two complexities : complexity of teneration and complexity of descritption. We do not find very clear the definitions he gives of these two concepts. 

- The « right measure » is a classical motto ; for instance, the use of symmetry in painting seen by [Funck-Hellet] : « Symmetry brings a voluntary order… but if, at first sight, the symmery is visible, it destroys the unity of aspect and downgrades the superior attractiveness of the composition » (our translation from French)

- A “right measure” of variation has been measured empirically in our [Roxame] software for image generation. It applies to pixelized images, and our experiences deal with 640x480 pixels. We define here “complexity” as the proportion of pixels who are different of the left and the upper ones. The best images have a complexity near to 2/3. Images of lower complexity appear to poor (with the limit case of monochromes, of course). Images of higher complexity (up to 100%) are “natural” images, like photographs, without “artistic” effect.

- A much more similar pattern is proposed by Langton, reproduced in  [Johnston, 2008] but we  could not find the original.

This figure is all the more interesting since it refers to whe Wolfram’s artificial life. But it does not give the coordinates of the cusp.  But it lets think that the IV class, the most seductive, is limited to a well defined value of <λ ; and that is certainly not the cas in this context.

A definition of this  <λ may be found in an article by Li, Langton et al.  Transition Phenomena in Cellular Automata Rule Space [Langton, 1990]

This definition has a very good plus : on the x axis, instead of the ill-defined complexity, it uses a measurable transition effect. Besides, playing on the genome level, and not on the resulting images (phenome), it could be used in generative algorithms (cellular automata, Markov chains, perhaps generative grammars).

An explanation of that
http://web.eecs.utk.edu/~mclennan/Classes/420-594-F07/handouts/Lecture-03.pdf

- Gaussian figures of complexity are  given in a synthetic paper by Pablo Funes (indicated to us by Samuel Monnier) “Complexity measures for complex systems and complex objects” [Funes]. This text provides interesting references on complexity, but nothing more preciste about coordinates.

Funes concludes evasively : “Effective complexity and total information emphasize the subjective nature of complexity as a relationship between natural phenomena and an interested observer. Complexity is more in the way that phenomena are observed (i.e., the model used) than in complicate elaborations made after the model has been imposed.” If we follow him, we should make models of the observation process, perhaps with a call to neurosciences.

- Samuel Monnier does not think possible to define a set of algorithms (what Galanter calls "Generative Art Systems") and hence a sufficiently precise definition of effective complexity. He writes (we translate) : « It “sems that this kind of effort be doomed to failure, if we consider all the algorithms. Indeed, a theorem ays that if the Kolmogorov complexity of an algorihm is higher than a diven number, it cannog be proven. In other words, the Kolmogorov complexity of most algorithms is not computable, ant is cannot even be located on the x axis. The explanation is given in [Wikipedia, Kolmogorov]. But this obstacle could perhaps be avoided if you limit the search to a finite set of algorithms.
“ Similar things could be done with images. For a given definition and bit depth, we have a finite number of possible images. Then the Kolmogorov complexity of an image can be defined as the size of the smallest program able to paint it. That would be the x axis of the Gell-Mann figure. Then you would have to define a natural notion of effective complexity on the y axis. If you have that, then you can hope to compare effective complexity with attractiveness for a spectator. But it appears clearly that the attractivity of an image cannot be related exclusively to any notion of  effective complexity” .

It is clear, anyway, that the attractivity cannot be defined without taking the spectator into account. But the spectator can be modeled, individually or collectively. That’s anyway the kind of things that does the Google ranking for documents.

Fig. 1. Energetic schema of evolution (first approximation). Ox, is the greater probability axis (entropy),  Oy is the  axis  (seemingly of less probability) : « orthogenesis » of biological growing complexity. The cosmic energy, not-ordered (tensed) in a, reaches a mximum or order in b, then becomes dis-orderes (distended) totally in c.

- It is the conflict that is the « effective complexity » for Eisenstein. (We translate from French, in [Albera]) : “In the domain of Art, the aesthetic principle of dynamics embodies itself in the conflict as the most essential principle of existence of any work of art and artistic genre.  The hypertrophy of an initiative conscious of its aim – of the rational logic principle – freezed art into a mathematical technicism (The landscape becomes a plane, “Saint Sebastian” an anatomic drawing). The hypertrophy of the organica natural – of the organic logic – dissolves art into shapelessness (Malevich becomes Kauflblach, Archipenko a waxworks museum).

Indeed,
- the limitum of the organic form (passive principle : be there) is nature,
- the limitum of the rational form (active production principe), and on the intersection of nature an industry, there stands art. “

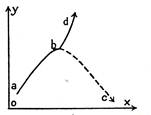
- A « mysterious b point » is seen by Teilhard de Chardin [Teilhard, 1952]. But he sketches a take off track, not explored by other thinkers, though not totally dissimilar to the drawing by Langton.

Fig.2.Energetic schema of evolution (second approximation). bd, escape branch for reflected energy, through b (superior critical point of convergence and reflection.

- Democracy can also be considered a sort of peak between dictature (monarchy) and anarchy.

- Decision processes could be mapped into Langton-like classes :

- Poetry is an important example for the search for this type of balance between order an disorder. “The function of verse is to give to the spoken language as much musical power as possible. This exceptional power is reached by adding (as the measure in music) an element of security for the ear and the mind, to the surprise element of ordinary language”. (our translation from  [Dorchain]). The role of transgression and its expressive effects is studied by [Leech, 1969].

- A lot of considerations of this type around Shannonian complexity  : for example [Moles, 1972], [McCormack, 2012] which includes communications by Galanter and Schmidhuber on this topic, and [Berger-Lioret 2012].

Resonance

(See some comments in Digital Universes

- Another track is suggested to us by the fact that art is the creation of an "unpublished resonance". Now, letting aside here the "unpublished" aspect, resonance is formally interesting And, as shown for example in [Wikipedia, resonance] resonance presents precisely a curve with a peak. Basically, resonance is given by a formula of the form : a/( d ** 2) + c, where a is a scaling parameter, d is the difference between the exciting signal and the resonator specific frequency and c a damping factor. When c is large, the peak is flattened (the resonator is lazy, but answers to a wide range of exciting signals). When c is small, the peak is acute (the resonator is sensitive only to frequencies near its own, but then can accumulate energy. In the limit case where c is null, the peak would reach the infinite.

That would bring an interesting forward jump in our reflexion, for two reasons :
- resonance takes us out of an purerly internal analysis of a work and its complexity up to an appreciation of a relation with something another… which could be the spectator ; here, the work becomes the energy loaded frequency with renders active the resonator ;
- we have a full quantitative and mathematical expression of the curve and of the peak (which is not a properly a cusp, so good for us also).

We can also observe that the formula is the inverse of a parabola. When d is null, we are at the base of the parabola with an interesting feature : on that point, there is no preferred direction, somehow the system is "free to chose". If we transform our flat parabola into a parabolic cup, then, at the bottom of the cup, the system is free of going on any direcdtion on a whole 360° gamut.

- An analogy with the border between two phases of matter is evoked by[ Bailly-Longo], but is also rather natural. For instance, variety of forms in general (waves, foam) as well as life, reaches a maxiwemum around the surface of water. Which is not point, but somehow “rounded”, like the resonance curves. Probably a rather similar formula could be proposed.

Benard Instability (Enseeiht document).

Its near the surface (and the heated bottom of the recipient, another phase change urface) that boiling moves are mainly observed. And so for the “dissipative structures”, like Bénard instabilities [Prigogine, 1971], [Enseeiht]. Another example of pleasant complexity arising on a phase shifting surface.

- An interesting question is asked by Cristian S. Calud and  J.P. Lewis ( still unpublished) : Is there a universal image generator. Or, more precisely, is there a generator able to produce all the images satisfying a given criterion. Perhaps this issue could profit of our peak images, if we interpret the criterion as a resonance performance.

References

Abel & Trevors : http://www.ncbi.nlm.nih.gov/pmc/articles/PMC1208958/, summing up a communication by Abel and Trevors, not accessible. They have also published several other papers, on neighbouring topics : http://www.tbiomed.com/content/4/1/47.

Albera François : Eisenstein et le constructivisme russe.  Lausanne. L’âge d’homme 1990.

Bailly Francis and Longo Giuseppe : Mathématiques et sciences de la nature. La singularité physique du vivant. Hermann 2006.

Berger Pierre and Lioret Alain : L’art génératif. L’Harmattan 2012.

Chauvet Gilbert : La vie dans la matière. Le rôle de l'espace en biologie. Flammarion 1995.

Dessalles Jean-Louis :  http://www.simplicitytheory.org/

Dorchain Auguste : L’art des vers, Garnier, Paris, 1933

Enseeiht : http://hmf.enseeiht.fr/travaux/CD0001/travaux/optmfn/hi/01pa/hyb72/bm/bm.htm

Funck-Hellet : http://diccan.com/Autres_auteurs/Funck-Hellet_texte.htm

Funes http://pages.cs.brandeis.edu/~pablo/complex.maker.html

Gell-Mann Murray :  Le quark et le jaguar．s.l.，Flammarion，<1997．

Johnston John : The Allure of Machinic Life．s.l.，MIT Press，<2008．

Langton Chris :  Transition Phenomena in Cellular Automata Rule Space . (published in  Physica,  1990) and downloadable from http://www.nslij-genetics.org/wli/pub/physicad90-no-figure.pdf. 

Leech Geoffrey N.  : A linguistic guide to English poetry. Longman 1969.

McCormack John and Mark d’Inverno, eds : Computers and Creativity. Springer 2012.

Moles Abraham : Théorie de l'information et perception esthétique. Denoël 1972.